SLOPE OF A LINE

-
Slope of a line

  • A line in a coordinate plane forms two angles with the x-axis, which are supplementary. 
  • The angle θ made by the line l with positive direction of x-axis and measured anti-clockwise is called the inclination of the line. Obviously 0° ≤ θ ≤ 180°.
  • We observe that line parallel to x-axis, or coinciding with x-axis, have inclination of 0°. 
  • The inclination of a vertical line ( parallel to or coinciding with y-axis ) is 90°.
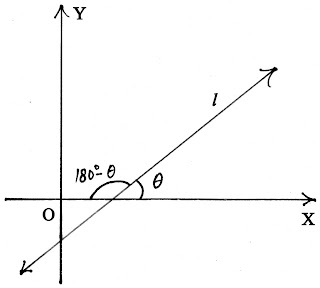
If θ is the inclination of a line l, then tanθ is called the slope or gradient of the line l. The slope of a line having inclination 90° 
is not defined.

 The slope of a line is denoted by m.

Thus m = tanθ, θ ≠ 90°.

It may be observed that slope of x-axis is zero and slope of y-axis is not defined.

Slope of a line when coordinates of any two points on the line are given 

Let P ( x, y ) and Q ( x, y ) be two points on a line l with inclination θ. Then slope is given by








Comments

Post a Comment

Popular posts from this blog

DOT PUZZLE ( CONNECT ALL THE DOTS WITHOUT LIFTING THE PEN AND WITH ONLY FOUR STRAIGHT LINES WITHOUT ANY OVERLAP )

-
Image
Here we would solve a dot puzzle Consider the figure below Connect all the dots without lifting the pen with only 4 straight lines and the lines do not overlap. Here are some examples already done for you but not been solved because some dots left to be crossed. You could see that some dots have been left to be crossed by the line. So do you think you could solve it. Let us try one more time. Now, what do you see, the puzzle has been solved with the required conditions. Find out some more solutions to this question.
Read more

EULER TRAIL PUZZLE

-
Image
What is an Euler trail ? An Euler trail ( Euler path ) is a trail in a finite graph which visits every edge exactly once. An Euler trail is a walk on the edges of a graph which uses each edge exactly once. Euler trail is also called an Euler chain , Euler walk , Euler path . Here is the puzzle as you could see in the picture. Your job is to cross each line exactly once without lifting your pen.  In the following figure consider every marked line as a single line and each must be crossed once without lifting you pen. Here are some examples already done, but in each case, one line is left ( marked red ) to be crossed . See the figures. Have you got the solution ? well, this is impossible to do however hard you try.  Explanation Consider the figure below and find the numbers of line reaching the centre of each box. In the figure you could see that there are three centre points with odd number o...
Read more