MATHEMATICS ENCYCLOPEDIA

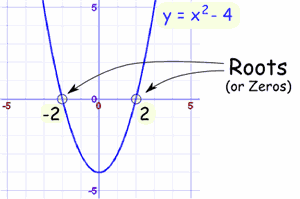
Oxford dictionary definition: An expression of more than two algebraic terms , especially the sum of several terms that contain different powers of the same variable(s ). Cambridge dictionary definition: A number or variable ( = mathematical symbol ), or the result of adding or subtracting two or more numbers or variables. A polynomial is an algebraic expression in which the variables have non-negative integers exponent only and involves the operations of addition, subtraction, multiplication. Examples are x^2 + 3x + 4,  4x^2 - 6x^2y^2 + x^3 + 7y^3 etc.  The zeroes of polynomial are precisely the x-coordinates of the points, where the graph of y = p(x) intersect the x-axis. The linear polynomial ax + b, a is not equal to zero, has exactly one zero. Quadratic polynomial can have either two distinct or two equal zeroes or no zero. This implies the polynomial of degree 2 has atmost 2 zeroes. Polynomial of degree 3 ( cubic polynomial ) can have atm...

Fundamental theorem of algebra states that every non-zero, single variable polynomial of degree n with complex cofficients has exactly n complex roots. Fundamental theorem of algebra is also stated as, every non-constant single-variable polynomial with complex cofficients has at least one complex roots. This also comprises polynomial with real cofficients because every real number is a complex number with imaginary part equal to zero.

Fundamental theorem of arithmetic is also called as unique factorization theorem or the unique prime factorization theorem. Definition of fundamental theorem of arithmetic: Every Integer  greater than 1 can be factored into a product of primes in exactly one way ( aside from rearranging the factors ). Example 24=2*2*2*3. The requirement of the factors to be prime is necessary. The theorem explains why 1 is not considered prime. If 1 would be prime, factorization would no longer be unique e.g. 4 = 2*2 = 2*2*1 = 2*2*1*1. This theorem explains that every integer greater than 1 is either prime or is the product of prime numbers, and the product is unique.

Real numbers are the collection of rational and irrational numbers. Real numbers can be represented using a real number line. In general natural numbers, whole numbers, integers, rational and irrational numbers, all are real numbers.  A complex number is a number of the form a+b i , where a and b are real numbers and i is the imaginary unit such that i ^2= -1 All the real numbers are complex numbers with imaginary part equal to zero.

Pime numbers : Positive integer greater than 1 that is divisible only by one and itself. Examples of prime numbers are 2,3,5,7,11 etc. Composite number: Positive integer having factors other than 1 and itself. In general, positive integer which is not prime is compostive. Examples are 4,6,8,10,12 etc.  0 and 1 are niether prime nor composite.

Even numbers: An even number is an integer divisible by two. Even numbers are either positive or negative. Zero is an even number. Odd numbers: An odd number is an integer not divisible by two. Odd numbers could be either positive or negative. 1 is the first positive odd number.

Irrational numbers can be defined as: A number is an irrational, if it has a non-terminating and non repeating decimal representation. An irrational number can't be written in the form p/q, where p and q are integers and q is not equal to zero. All the square root of natural numbers are irrational other than perfect squares.

Definition of rational numbers: Numbers that can be written in the form p/q where p and q are integers and q is not equal to zero. We can write any natural number, whole number and integers as p/q with q =1. Hence they are all rational numbers, e.g. 2=2/1, -7= ( -7 )/1 etc. Rational numbers have either terminating or non-terminating but repeating ( recurring ) decimal representation.

Numbers can be classified into following: Natural numbers ( N ): The numbers used in counting are called the natural numbers. the numbers are { 1,2,3, ........}. Whole numbers ( W ): The counting numbers including zero are whole numbers. The numbers are { 0,1,2,3, ........}. Integers ( Z ): Postive and negative naturals numbers are integers. The numbers are { ..... ,-3, -2, -1, 0, 1, 2, 3, ...... }. Rational numbers ( Q ): The numbers that can be expressed as as a ratio of an integer to a non-zero integer are rational numbers. Rational numbers are of the form p/q and q may be equal to 1, you can say that every integer is a rational number but every rational is not integer e.g. 5/3 is is rational but not integer. Irrational numbers ( II ): The numbers that are not expressible as a ratio of two integers , and having an infinite and non-recurring expansion when expressed as a decimal. Examples of irrational numbers are π, Eular's number ( e ), square root of 2 etc. All the...

The number is a concept, the numeral is the way we use to write it. A number may be expressed in many different ways using different numerals. But each numeral will always represent the same number e.g. three can be written as 3, iii, or III but it represent the same number three. For any number there can be several numerals ,but a number is just one numerical value. 

Number can be defined in several ways such as: Oxford dictionary definition: An arithmetic value, expressed by a word, symbol, or figure representing a particular quantity and used in counting and making calculations. Cambridge dictionary definition: A sign or symbol representing a unit that forms part of the system of counting and calculating.

There is no general accepted definition of Mathematics. There are many definitions of Mathematics, some of them are as follows: Cambridge dictionary definition: The study of numbers, shapes, and space using reason and usually a special system of symbols and rules for organizing them. Oxford dictionary definition: The abstract science of numbers, quantity , and space, either as abstract concepts ( pure Mathematics ), or as applied to other disciplines such as physics and engineering ( applied Mathematics ).

The blog comprises contents related to Mathematics. It includes the very basic concepts like definition of Mathematics, types of numbers etc. as well as higher Mathematics like Calculus, hypothesis, conjectures etc.         The subscriber on the blog could send their Mathematics related papers ( either hand written or digital ). If found interesting and useful will be published with their names. Find new post every week . Don't forget to subscribe.