MATHEMATICS ENCYCLOPEDIA

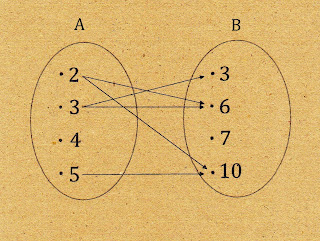
Function Function is a special type of relation. It is one of the most important concepts in mathematics.The word function is derived from a Latin word meaning operation and the word mapping and map are synonimous to it. Functions play very important role in Calculus. A relation f from a set A to a set B is said to be a function if every element of set A has one and only one image in set B. In other words, a function f is a relation from a non-empty set A to a non-empty set B such that the domain of f is A and no two distinct ordered pairs in f   have the same first element . If f is a function from from A to B and ( a, b ) ∈ f , then f  ( a )  = b, where b is called the image of a under f and a is called the preimage of b under f . The function f  from A to B is denoted by f  : A ⟶ B. A function which has either R ( real numbers ) or one of its subsets as its range is called a real valued function. Further, if its domain is also either R ( rea...

Relations A relation R from a non-empty set A to a non-empty set B is a subset of the cartesian product         A Х B . The subset is derived by describing a relationship between the first element and the second element of the ordered pairs in A Х B. The second element is called the image of the first element. The set of all first elements of the ordered pairs in a relation R from a set A to a set B is called the domain of the relation R. The set of all second elements in a relation R from a set A to a set B is called the range of the relation R. The whole set B is called the codomain of the relation R.  Range ⊆ codomain . Example Let A = { 2, 3, 4, 5 } and B = { 3, 6, 7, 10 } . A relation R from set A to the set B as follows: R = { ( x, y ) : x divides y, where x ∈ A and y ∈ B }. We find that 2 divides 6 and 10, 3 divides 3 and 6, 5 divides 10 and there is no number in B that can be divided by 4. Thus ( 2, 6 ) ∈ R, ( 2, 10 ) ∈ R, ( 3, 3...