LINEAR INEQUALITIES AND ITS REPRESENTATION

-
Definition of linear inequality
 A statement involving two real numbers or two algebraic expressions related by the symbol ' < ', ' > ', ' ≤ ', ' ≥ ' form an inequality.

Types of inequalities

  • Numerical inequalities, e.g. 7 > 5, 11 < 8 etc.
  • Literal inequalities, e.g. x < 7, y > 4, x ≥  6 etc.
  • Double inequalities, e.g. 3 ≤ x < 5, 6 < y < 11 etc.
  • Strict inequalities, e.g. x < 7, ax + by > 0 etc.
  • Slack inequalities, e.g. ax + by ≤ 0
  • Quadratic inequalities, e.g. ax^2 + bx + c > 0 etc.
Rules for solving an inequality

  • Rule 1: Equal numbers may be added to ( or subtracted from ) both sides of an inequality without affecting the sign of inequality.
  • Rule 2: Both sides of an inequality can be multiplied ( or divided ) by the same positive number. But when both sides are multiplied or divided by a negative number, then the sign of inequality is reversed.
Representation of inequality on real number line

LINEAR INEQUALITIES AND ITS REPRESENTATION

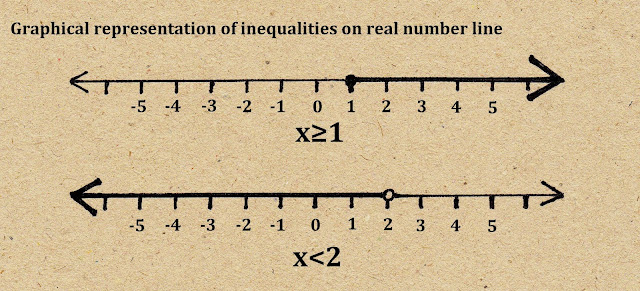
Comments

Post a Comment

Popular posts from this blog

DOT PUZZLE ( CONNECT ALL THE DOTS WITHOUT LIFTING THE PEN AND WITH ONLY FOUR STRAIGHT LINES WITHOUT ANY OVERLAP )

-
Image
Here we would solve a dot puzzle Consider the figure below Connect all the dots without lifting the pen with only 4 straight lines and the lines do not overlap. Here are some examples already done for you but not been solved because some dots left to be crossed. You could see that some dots have been left to be crossed by the line. So do you think you could solve it. Let us try one more time. Now, what do you see, the puzzle has been solved with the required conditions. Find out some more solutions to this question.
Read more

EULER TRAIL PUZZLE

-
Image
What is an Euler trail ? An Euler trail ( Euler path ) is a trail in a finite graph which visits every edge exactly once. An Euler trail is a walk on the edges of a graph which uses each edge exactly once. Euler trail is also called an Euler chain , Euler walk , Euler path . Here is the puzzle as you could see in the picture. Your job is to cross each line exactly once without lifting your pen.  In the following figure consider every marked line as a single line and each must be crossed once without lifting you pen. Here are some examples already done, but in each case, one line is left ( marked red ) to be crossed . See the figures. Have you got the solution ? well, this is impossible to do however hard you try.  Explanation Consider the figure below and find the numbers of line reaching the centre of each box. In the figure you could see that there are three centre points with odd number o...
Read more