LAW OF SINES AND COSINES AND OTHER APPLICATIONS OF TRIGONOMETRY

-
Solution of triangles and trigonometry applications.

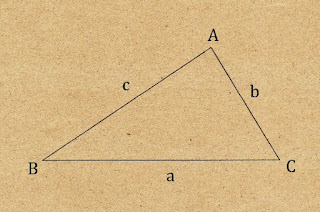
In a triangle ABC, the angles are denoted by the capital letters A, B, C and the lengths of sides opposite to these angles are denoted by a, b, c respectively. See the figure.
LAW OF SINES AND COSINES AND OTHER APPLICATIONS OF TRIGONOMETRY












Some important formulae regarding the sides and angles of a triangle are given as follows:



The law of  sines: In any triangle ABC




where R is the radius of the circumcircle of the triangle ABC. R is also known as the circumradius of the triangle.


The law of  cosines: In any triangle ABC

The law of cosines

Trigonometric ratios of half-angles in terms of the sides

Let 2s = a + b + c, so that s is the semi-perimeter of triangle ABC. Then,

Trigonometric ratios of half-angles in terms of the sides

Napier's anology: In any triangle ABC

Napier's anology











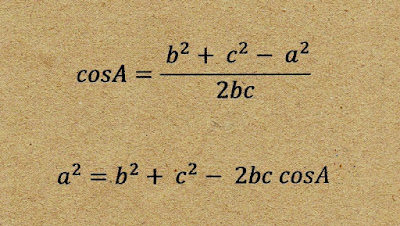
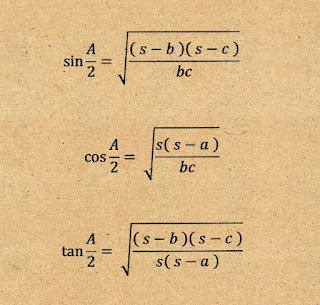
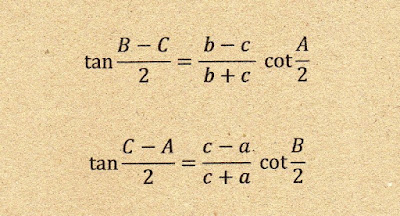
Comments

Post a Comment

Popular posts from this blog

DOT PUZZLE ( CONNECT ALL THE DOTS WITHOUT LIFTING THE PEN AND WITH ONLY FOUR STRAIGHT LINES WITHOUT ANY OVERLAP )

-
Image
Here we would solve a dot puzzle Consider the figure below Connect all the dots without lifting the pen with only 4 straight lines and the lines do not overlap. Here are some examples already done for you but not been solved because some dots left to be crossed. You could see that some dots have been left to be crossed by the line. So do you think you could solve it. Let us try one more time. Now, what do you see, the puzzle has been solved with the required conditions. Find out some more solutions to this question.
Read more

EULER TRAIL PUZZLE

-
Image
What is an Euler trail ? An Euler trail ( Euler path ) is a trail in a finite graph which visits every edge exactly once. An Euler trail is a walk on the edges of a graph which uses each edge exactly once. Euler trail is also called an Euler chain , Euler walk , Euler path . Here is the puzzle as you could see in the picture. Your job is to cross each line exactly once without lifting your pen.  In the following figure consider every marked line as a single line and each must be crossed once without lifting you pen. Here are some examples already done, but in each case, one line is left ( marked red ) to be crossed . See the figures. Have you got the solution ? well, this is impossible to do however hard you try.  Explanation Consider the figure below and find the numbers of line reaching the centre of each box. In the figure you could see that there are three centre points with odd number o...
Read more